How to Solve an Absolute Value Inequality?
Introduction
The absolute values inequalities can be solved according to the inequality sign involved in the expressions. In this article, you will learn to solve absolute values inequaties by using just four steps.
How do you calculate the absolute value?
The absolute value of an inequality equation converts it into two parts i.e. writing the equation with greater than > and with less than < sign. To solve equations containing absolute value inequalities, following steps can be performed to solve inequalities.
- You have to remember that the absolute value inequality has to be solved for the values of variables involved like double absolute value inequality.
- Isolate the absolute value on one side and the constant on the other side.
- Write the equation in compound inequality by using greater than and less than signs. The inequality sign will vary according to positive and negative signs.
- Find the values of the variable x and write them on the number line.
Let’s see the following examples to understand how to solve absolute value inequalities equations.
Absolute Values Inequalities Example no. 1
Solve, 4 |x + 3| -7 ≤ 5.
To solve the given equation, first step is to convert it in isolated form as
4 |x + 3| - 7 ≤ 5
4 |x + 3| ≤ 5 + 7
4 |x + 3| ≤ 12
Dividing by 4 on both sides,
|x + 3| ≤ 3
In step two, rewriting the absolute value inequality as a compound inequality,
-3 ≤ x + 3 ≤ 3
Or it can be also be written as

x + 3 ≤ 3 and x + 3 ≥ -3
x ≤ 0 and x ≥ -6
Now shading the solution on the number line to represent it.
Absolute Value Inequalities Example no. 2
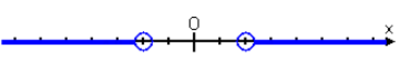
Solve |x| > 2 and express on the number line.
The given equation is already in isolated form. So,
x > 2 and x < -2
Expressing the solution of x on number line,
Example#3
Solve |2x + 3| < 6 and express on the number line.
The given equation is already in isolated form. So,
2x + 3 > 6 and 2x + 3< -6
Solving them for x, we get
x > 3 / 2 and x < -9 / 2
Shading the solution on number line,
Example#4
Solve |x + 2| < 3 and express on the number line.
The given equation is already in isolated form. So,
x + 2 > 3 and x + 2 < - 3
Solving them for x, we get
x > 1 and x < -5
Now graph the values of x,

Related:How to solve Double Absolute Value Inequalities? Examples
How to Solve Absolute Value Inequalities on a Calculator?
It is an easy and fast way to solve absolute inequalities by using an absolute value inequality calculator. It is because, here, you don’t need to solve inequality equations manually. Follow the given steps to find absolute value for inequality equations.
- Enter the values of constants b, c and d along with +, - signs.
- Or try the “Load Example” option that automatically fills the blanks with random values of b, c and d.
- Select the inequality sign, <, ≤ or ≥.
- Now simply click on the calculate button.
You will get the solution of the inequality equation with the respective given values and inequality sign.
FAQ’s
What is Inequality Involving Absolute Value?
The absolute value of an inequality equation is an algebraic expression that involves inequality symbols.
Can an Absolute Value of Inequality have one Solution?
When there is a zero in the right side of the isolated form, it implies that it has only one solution.
What are the 4 Steps to Solving an Absolute Value Equation?
The four steps to solve an absolute value inequality are
- Convert the equation in an isolated form.
- Writing the equation in compound inequality.
- Solve the compound inequality equation for the value of x.
- Graph the solution on the number line.